

It is represented by the formula a_n = a_(n-1) + a_(n-2), where a_1 = 1 and a_2 = 1. A Fibonacci sequence is a sequence of numbers in which each term is the sum of the previous two terms.The common ratio is obtained by dividing the current term by the previous term. It is represented by the formula a_n = a_1 * r^(n-1), where a_1 is the first term of the sequence, a_n is the nth term of the sequence, and r is the common ratio. Explicit Formula - from Wolfram MathWorld Number Theory Prime Numbers Prime Number Theorem Number Theory Number Theoretic Functions Number Theoretic Sums Number Theory Number Theoretic Functions General Number Theoretic Functions More. A geometric sequence is a sequence of numbers in which each term is obtained by multiplying the previous term by a fixed number.You can also add, subtraction, multiply, and divide and complete any arithmetic you need. The Math Calculator will evaluate your problem down to a final solution. It is represented by the formula a_n = a_1 + (n-1)d, where a_1 is the first term of the sequence, a_n is the nth term of the sequence, and d is the common difference, which is obtained by subtracting the previous term from the current term. Step 1: Enter the expression you want to evaluate.
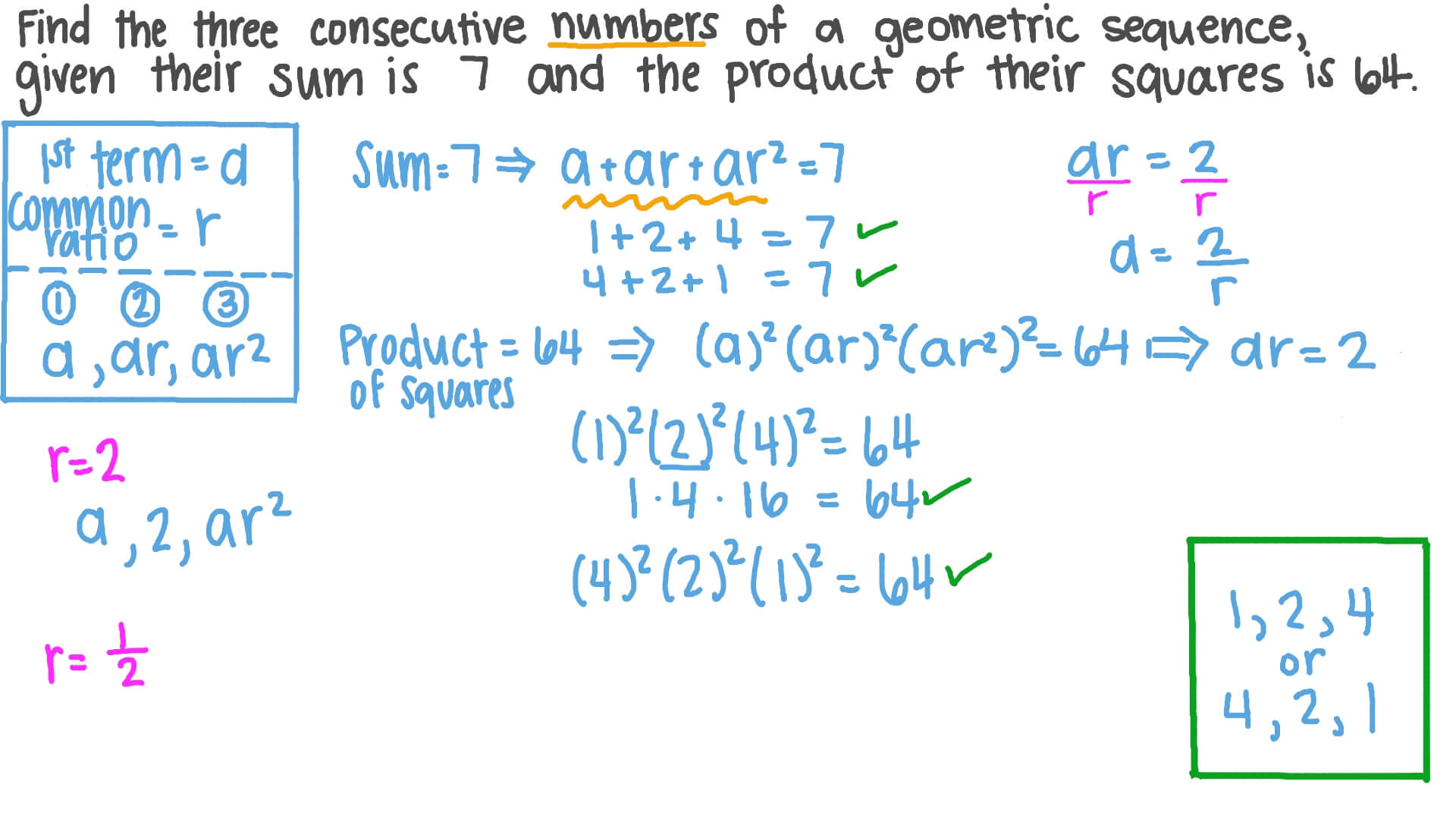
An arithmetic sequence is a sequence of numbers in which each term is obtained by adding a fixed number to the previous term.The most common types of sequences include the arithmetic sequences, geometric sequences, and Fibonacci sequences.The formula for the nth term of a Fibonacci sequence is a_n = a_(n-1) + a_(n-2), where a_1 = 1 and a_2 = 1.


 0 kommentar(er)
0 kommentar(er)
